Exercise F-6.1.4 TechMech 2 - Mechanics of Materials

Practice Exercise F-6.1.4

Second Moment of Inertia: Determine the Axial and Biaxial Area Moment of Inertia

Problem Statement

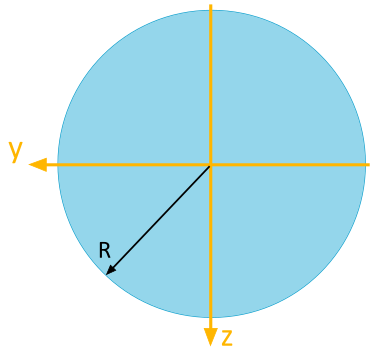
Circular area for which the moment of inertia (axial, biaxial) is to be calculated. Radius: R, Center: y, z, y positive to the left, z positive downward. — Fig. 1: Circle Area with given Principal Axes

For the circular area depicted, the following area moments of inertia are to be determined with respect to the illustrated $y$, $z$-coordinate system:

Axial Area Moment of Inertia $I_y$
Axial Area Moment of Inertia $I_z$
Biaxial Area Moment of Inertia $I_{yz}$

Short Solution

a) Determine the Axial Area Moment of Inertia $I_y$

$$ \begin{aligned} I_y&= \dfrac{1}{4}R^4\pi \end{aligned} $$

b) Determine the Axial Area Moment of Inertia $I_z$

$$ \begin{aligned} I_z &= \dfrac{1}{4}R^4\pi \end{aligned} $$

c) Determine the Biaxial Area Moment of Inertia $I_{yz}$

$$ \begin{aligned} I_{yz} &= 0 \end{aligned} $$

Comprehensive Solution

One approach to determine the area moments of inertia is to perform the calculation in polar coordinates.

To apply the calculation method, it is necessary to determine the functional relationship $r(\varphi)$.

We find this by illustrating the relationship between any angle $\varphi$ and the corresponding radius $r(\varphi)$ of the circle: The radius is constant. This property of the circle simplifies the calculation using this method. Therefore, it is preferable to Cartesian coordinate methods when calculating circular areas.

Continue with TechMechAcademy+

Everything. Always. Everywhere.

With TechMechAcademy+ full access to all content.

Become a TechMechAcademy+ Premium Member!

Are you already a TechMechAcademy+ premium member? Then please log in here to enjoy full access to all content.